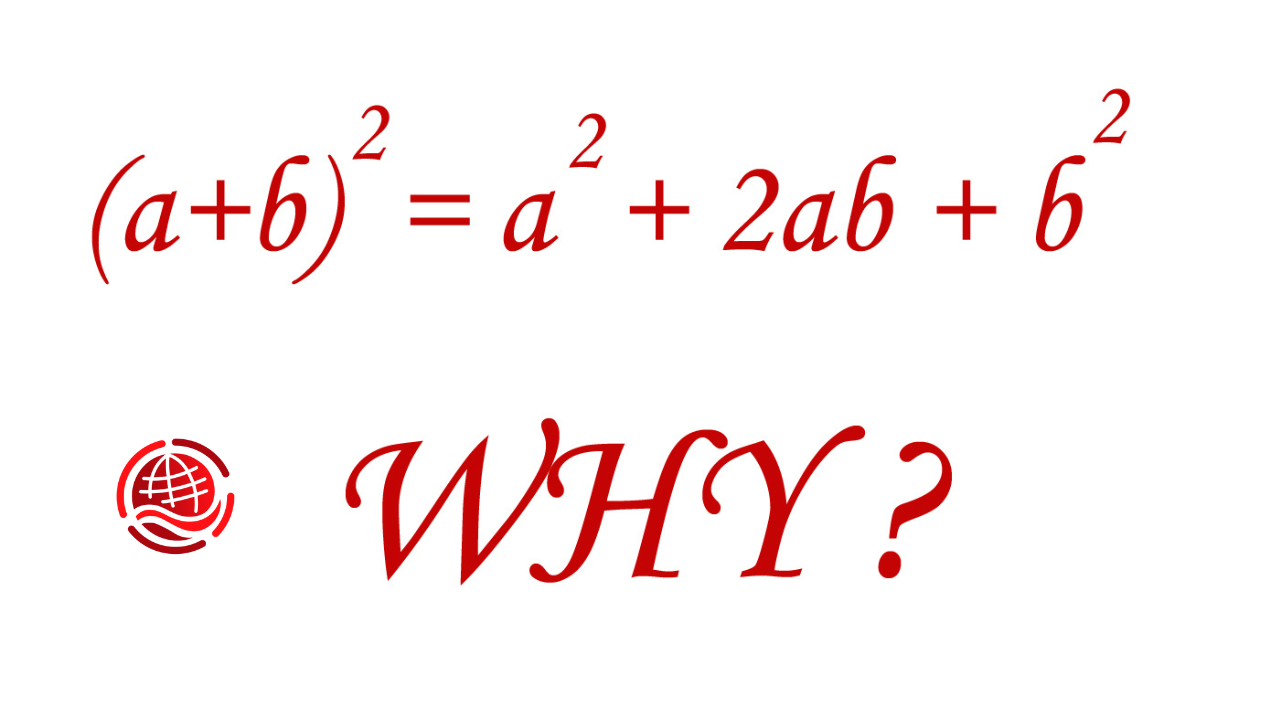The (a+b)^2 formula, also known as the square of a binomial formula, is a fundamental concept in algebra. This formula is used to find the square of the sum of two numbers, a and b. Understanding and mastering this formula is essential for solving various algebraic equations and factorizing trinomials. In this blog post, we will delve into the (a + b)2 formula, its geometric interpretation, practical applications, solved examples, and frequently asked questions.
Understanding the (a+b)^2 Formula
At its core, the (a+b)^2 formula exemplifies a fundamental principle in algebra, focusing on the square of the sum of two terms, a and b. This identity is expressed mathematically as (a + b) 2 = a2 + 2ab + b2. The derivation of this formula begins by multiplying the binomial (a + b) by itself, leading to an expansion that reveals the intricate relationship between the individual squares of a and b and their product.
To fully appreciate the mechanics of this formula, let’s dissect its derivation step by step. Initially, we consider the multiplication of the binomial with itself: (a + b)(a + b). Applying the distributive property—where each term inside the first parentheses is multiplied by each term inside the second—we obtain a^2 + ab + ba + b^2. Observing the terms ab and ba as identical, we combine them to yield 2ab, arriving at the simplified and widely recognized form of the formula: a^2 + 2ab + b^2.
This algebraic identity is not just a mere mathematical curiosity; it is a powerful tool in simplifying and solving algebraic expressions. Its significance is particularly evident when dealing with polynomial equations, where recognizing the pattern a^2 + 2ab + b^2 allows for quick factorization or expansion. Furthermore, this formula serves as a bridge connecting algebraic expressions with geometric interpretations, thereby enhancing our conceptual understanding of algebraic operations through visual means.
In essence, the (a + b)^2 formula encapsulates a fundamental algebraic concept that transcends its apparent simplicity. By understanding how to derive and apply this formula, learners can unlock a deeper comprehension of algebraic expressions, laying the groundwork for more advanced mathematical explorations. Through the manipulation and application of this formula, we gain not only the ability to simplify and solve equations but also a richer, more connected understanding of the mathematical world.
Geometric Interpretation of the (a+b)^2 Formula
Geometric interpretation adds a vivid dimension to understanding mathematical concepts, and this is particularly true for the (a + b)^2 formula. The beauty of this algebraic identity can be seen through a simple yet illustrative geometric proof that bridges abstract algebra with tangible visualizations. Imagine constructing a large square, the sides of which measure (a + b) in length. This square is then divided into smaller geometric shapes: two smaller squares and two rectangles. The side of one small square measures ‘a’ units in length, and the other ‘b’ units correspond to the terms in the binomial we are squaring.
These geometric shapes represent the individual components of the (a + b)^2 formula. The area of the first square, which is ‘a’ by ‘a’, gives us a^2, while the area of the second square, ‘b’ by ‘b’, gives us b^2. The rectangles, each sharing sides of lengths ‘a’ and ‘b’, contribute areas of ab each. When these areas are combined—adding up the areas of the two squares and the two rectangles—we obtain the total area of the large square, which is expressed algebraically as a^2 + 2ab + b^2.
This geometric approach not only validates the algebraic formula but also provides a visual understanding of how the terms of the binomial square contribute to the total area. It elucidates the concept that the square of the sum of two numbers encompasses not only the individual squares of these numbers but also twice the product of the two numbers, represented by the area of the rectangles. By visualizing these geometric relationships, learners can more deeply comprehend and internalize the (a + b)^2 formula, making it a powerful tool in both algebraic and geometric contexts.
Practical Applications of the (a+b)^2 Formula
The practicality of the (a + b)^2 formula extends far beyond textbook exercises, impacting various fields and real-world scenarios. This algebraic identity plays a crucial role in simplifying complex algebraic expressions, thus facilitating smoother problem-solving in mathematics and its applications. One of the notable uses of this formula is in the realm of polynomial algebra, where it assists in the quick factorization of specific trinomial forms, namely a^2 + 2ab + b^2. By recognizing this pattern, mathematicians and students alike can efficiently decompose complex expressions into simpler, more manageable components.
In addition to factorization, the (a + b)^2 formula is indispensable in the expansion of binomials. This application is particularly useful in higher-level algebra, where it becomes necessary to manipulate and expand expressions for further analysis or simplification. Through its application, learners develop a deeper understanding of the structure of algebraic expressions and enhance their problem-solving strategies.
The formula’s utility is also evident in the domain of quadratic equations, where it can be employed to rewrite equations in a form that is more conducive to solving through methods such as completing the square or applying the quadratic formula. Furthermore, this algebraic identity finds its place in the proof of various mathematical theorems and identities, serving as a foundational element in the demonstration of more complex mathematical concepts.
Beyond the confines of pure mathematics, the (a+b)^2 formula has applications in physics, engineering, and economics, where it aids in the modelling and solving of problems related to these disciplines. From calculating areas in geometry to analyzing profit functions in economics, the versatility and practicality of this formula underscore its importance across a broad spectrum of academic and professional fields. Its widespread application highlights not only the interconnectedness of mathematical concepts but also the formula’s integral role in fostering analytical thinking and problem-solving skills.
Solved Examples Using the (a+b)^2 Formula
Diving into the practical applications of the (a + b)^2 formula, let’s explore its versatility through a series of solved examples. These instances demonstrate not just the formula’s utility in algebraic manipulation but also its role in enhancing problem-solving techniques across various mathematical contexts.
Example 1: Determine the value of (3x + 2y)^2.
Applying the formula directly, we identify an as 3x and b as 2y. Substituting these values into the (a + b)^2 formula gives us:
(3x + 2y)^2 = (3x)^2 + 2(3x)(2y) + (2y)^2
Simplifying the expression yields 9x^2 + 12xy + 4y^2. This illustrates how the formula can quickly expand binomials into trinomials.
Example 2: Factorize x^2 + 4xy + 4y^2.
In this scenario, we observe that the given expression fits the pattern a^2 + 2ab + b^2, signalling that it can be directly factorized using the (a + b)^2 formula. By comparing, we find a = x and b = 2y, leading to the factorized form:
x^2 + 4xy + 4y^2 = (x + 2y)^2.
This example underscores the formula’s ability to streamline the factorization process, converting complex trinomials back into simpler binomial squares.
Example 3: Simplify (7x + 4y)^2.
To simplify, we recognize an as 7x and b as 4y. Substituting these into our formula, we compute:
(7x + 4y)^2 = (7x)^2 + 2(7x)(4y) + (4y)^2
Resulting in 49x^2 + 56xy + 16y^2. This computation demonstrates the formula’s effectiveness in expanding binomials, providing a clear path to simplification.
Through these examples, the (a + b)^2 formula reveals itself as a powerful algebraic tool capable of simplifying complex expressions, factorizing trinomials, and expanding binomials with efficiency and precision.
FAQs on the (a+b)^2 Formula
Frequently asked questions about the (a+b)^2 formula highlight its significance and applications in mathematical problem-solving. This section addresses some of the common inquiries that arise when exploring this foundational algebraic identity.
1. Can the (a + b)^2 formula assist in algebra beyond simple squaring?
Yes, beyond calculating the square of a binomial, this formula is instrumental in decomposing complex trinomials into simpler forms for easy factorization. It serves as a bridge in connecting different algebraic concepts and solving quadratic equations more efficiently.
2. Is there a visual method to understand the (a + b)^2 formula better?
Absolutely. By constructing a large square divided into smaller geometric shapes—two squares and two rectangles—one can visually comprehend how the formula encapsulates the sum of their areas (a2 + 2ab + b2). This geometric representation not only validates the formula but also enhances our grasp of algebra through spatial understanding.
3. Where does the (a + b)^2 formula find utility outside of classroom algebra?
This formula’s utility extends into various fields, including physics for calculating motion, engineering for structural analysis, and economics for modelling financial equations. Its application in simplifying expressions and solving equations proves invaluable in both academic studies and professional practices.
4. How does the (a + b)^2 formula aid in mathematical proofs?
Mathematicians often employ this algebraic identity to prove more intricate theorems and identities. It lays the groundwork for a deeper exploration of algebra and geometry, demonstrating the interconnectedness of different mathematical areas.
Read More: Demystifying Arithmetic Progression: The Essential Maths Sequence