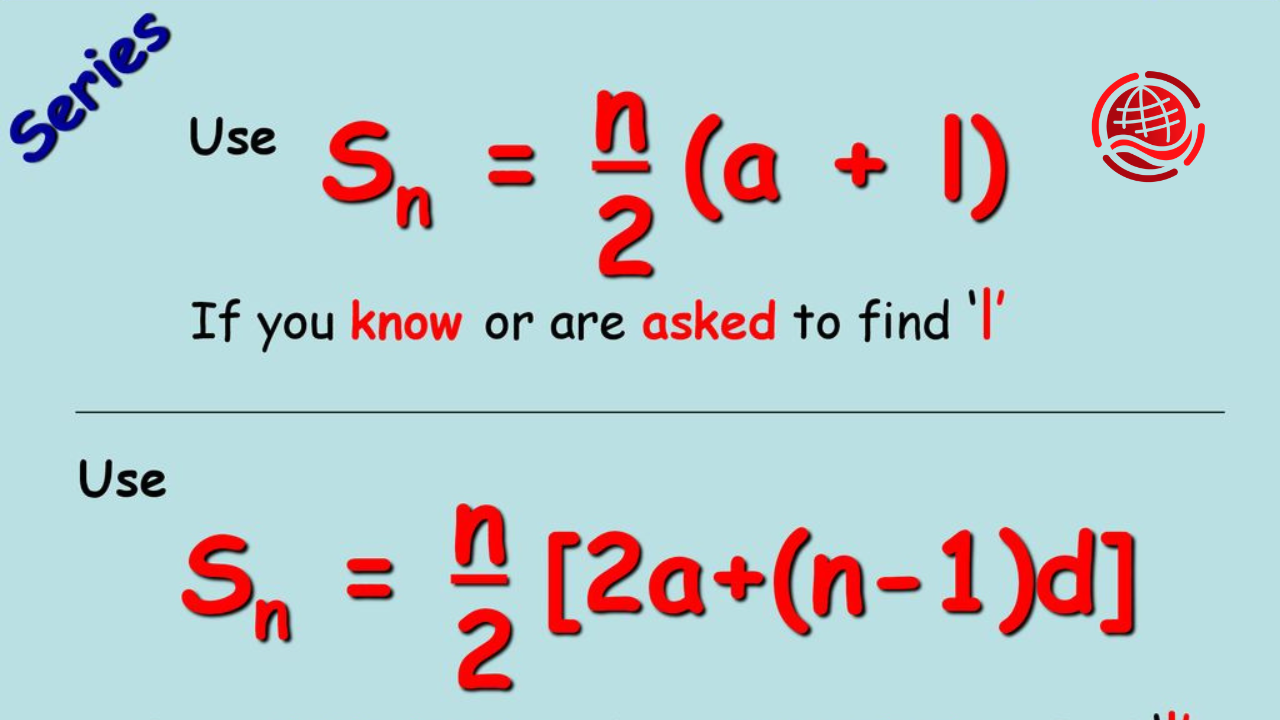Arithmetic Progression (AP) is a fundamental mathematical concept involving a sequence of numbers where the difference between consecutive terms is constant. This type of sequence is widely used in various mathematical calculations and formulas. One key formula in AP is s=n/2 2a+(n-1)d, which helps find the Sum of N Terms of AP. In this blog post, we will delve deeper into the world of AP, understanding its components, formulas, and practical applications.
Understanding Arithmetic Progression
Arithmetic Progression is a distinctive concept within mathematical sequences due to its unique characteristic – the constant difference between successive terms, known as the common difference (“d”). This attribute defines AP and differentiates it from other progressions like Geometric and Harmonic Progressions.
A simple example of this concept is the sequence 2, 5, 8, 11…, where the common difference is 3. Such a sequence demonstrates the linear nature of AP, where each term builds upon the last with a uniform increment. The simplicity and predictability of this pattern make AP a fundamental topic in mathematics, offering a straightforward approach to understanding sequences and series.
This progression’s practical applications span across various academic and real-world scenarios, underscoring its importance. Through AP, learners grasp the foundational principles of sequence and series, paving the way for more complex mathematical concepts.
Identifying the First Term and Common Difference
In any Arithmetic Progression, the first term, “a,” marks the starting point of the sequence, and the “common difference,” d, defines the uniform gap between any two consecutive numbers. These two parameters are pivotal for the construction and analysis of an AP. The first term sets the stage, offering a clear reference point from which the progression unfolds. Meanwhile, the common difference is the consistent additive factor that connects each term to its predecessor, creating a linear pattern that is easy to follow and predict.
Understanding these elements allows for exploring deeper aspects of the sequence, such as its growth direction, rate of increase or decrease, and overall structure. It is crucial to grasp these concepts early on, as they form the backbone of Arithmetic Progression and are essential for navigating through more complex problems and applications within the topic. Identifying these fundamental components unlocks the ability to analyze and construct any AP efficiently.
The General Form of an Arithmetic Progression
The essence of an Arithmetic Progression (AP) lies in its structured pattern, delineated by the equation a, a + d, a + 2d, a + 3d, and so forth. Here, “a” symbolizes the initial term of the series, setting the progression’s starting value, while “d” represents the common difference, indicating the consistent interval between each successive term. This formula acts as a blueprint, enabling the precise calculation of any term within the sequence by incorporating its position (n) into the framework.
It highlights the linear nature of AP, where each subsequent term results from adding a common difference to its predecessor. This pattern ensures a straightforward progression from one term to the next, making it easier to predict future terms or backtrack to prior ones within the sequence. By understanding this general form, learners can navigate through the intricacies of Arithmetic Progression with greater ease, applying this knowledge to solve various mathematical problems and explore the extensive applications of AP in real-world contexts.
Key Formulas in Arithmetic Progression
In Arithmetic Progression, the formulation of the nth term and the calculation of the sum of the first n terms are achieved through two pivotal formulas. To pinpoint any term within the sequence, the formula an = a + (n-1)d is utilized, where “a” represents the initial term, “d” is a common difference, and “n” is the term’s position.
This allows for precise identification of any term’s value within the progression. Conversely, when the objective is to ascertain the aggregate of the first n terms, the formula s = n/2[2a + (n-1)d] comes into play. This equation facilitates the summation process and underscores the sequence’s linear growth by incorporating the first term, common difference, and term count into the calculation. Both formulas are integral to understanding and applying the concepts of Arithmetic Progression, offering a structured approach to dissect and engage with this fundamental sequence.
Through these expressions, one can navigate the arithmetic progression landscape, making informed predictions and calculations critical for various mathematical explorations and applications.
Finite and Infinite Arithmetic Progressions
Arithmetic Progressions are broadly categorized based on the number of terms they encompass. Finite APs are characterized by a set number of terms, clearly defining the start and end of the sequence. This specific endpoint allows for applying formulas to calculate the sum of terms or identify a term’s position within the sequence.
Conversely, infinite APs extend indefinitely, lacking a definitive last term. This absence of an endpoint opens up intriguing mathematical explorations but also introduces complexities in terms of sum calculation and term identification, as traditional formulas for sum require adjustments to accommodate the infinite nature of the sequence.
Understanding whether an AP is finite or infinite is essential for applying the correct mathematical approach and appreciating the scope of problems each type can present. This distinction between finite and infinite sequences enriches our understanding of Arithmetic Progressions. It highlights the diversity within mathematical sequences and their application to problem-solving in both theoretical and practical contexts.
Sum of N Terms of AP Explained
Unlocking the calculation of an Arithmetic Progression’s sum for the initial n terms is made intuitive with the pivotal formula s = n/2[2a + (n-1)d]. This mathematical expression is a gateway to understanding how to aggregate a sequence’s values efficiently.
Here, ‘s’ denotes the sum we seek, ‘n’ signifies the count of terms to be summed, ‘a’ is identified as the sequence’s first term, and ‘d’ represents the uniform difference between consecutive terms. By inputting these specific values into the formula, the sum of any portion of an AP becomes readily calculable. This formula is particularly useful for sequences where direct addition becomes impractical due to many terms.
It also highlights the linear relationship within the progression, showcasing how the sum is directly proportional to the number of terms involved. This linear aspect ensures that the sum increases at a predictable rate as we increase the number of terms, providing a clear understanding of the sequence’s growth pattern. Implementing this formula, we can swiftly navigate through the complexities of AP, making it possible to solve problems related to cumulative values without the need for laborious addition.
Practical Examples and Solved Problems
Diving into practical examples and solved problems allows us to bridge the gap between theoretical knowledge of Arithmetic Progression (AP) and its application to tangible situations. For instance, imagine we’re tasked with finding the 20th term in the AP sequence of 3, 6, 9, 12, … Here, we quickly recognize that the first term, a, is 3 and the common difference, d, is 3. Applying the formula for the nth term, an = a + (n-1)d, we find that the 20th term, a20 = 3 + (20-1)3 = 3 + 57 = 60, revealing how seamlessly these formulas integrate into problem-solving.
Another example might involve calculating the sum of the first 50 terms of the AP sequence beginning with 4 and having a common difference of 2. Utilizing the sum formula, S = n/2[2a + (n-1)d], we plug in the values to get S50 = 50/2[2*4 + (50-1)2] = 25[8 + 98] = 25*106 = 2650. This calculation underscores the efficiency of the AP formulas in handling more substantial sequences where manual summation would be exhaustive.
Through these examples, we can observe the practical utility of AP in simplifying complex problems, making it an invaluable tool for mathematicians, scientists, and educators alike. Engaging with these problems enhances our comprehension and enables us to appreciate the elegance and applicability of Arithmetic Progression in everyday mathematical challenges.
Practice Problems on AP
Engaging with a variety of practice questions is crucial to solidify your grasp of Arithmetic Progression and enhance your problem-solving abilities. These exercises will help you apply the formulas for the nth term and the sum of n terms, deepening your understanding of AP’s fundamental principles. Consider trying your hand at calculating specific terms within an AP, summing a series of terms, and deducing the common difference given certain elements of the sequence.
For instance, attempt to find the 25th term of an AP with a first term of 5 and a common difference of 3. Or, calculate the sum of the first 20 terms of an AP where the first term is 2 and the common difference is 4. Another valuable exercise is determining the common difference when an AP’s 10th and 15th terms are known. These varied problems will challenge your comprehension and application of AP, fostering a more intuitive understanding of this critical mathematical concept. Engage with these problems to refine your mathematical skills and become proficient in navigating the intricacies of Arithmetic Progressions.
Read More: Understanding the Power of Newton’s Second Law: F=ma